GRE® Quant Practice | Solid Geometry

This GRE quant practice question is a geometry - solid geometry problem solving question. Computing the volume of a right circular cone obtained by recasting or rotating a sector of a circle.

Question 1 : A sector of a circle of radius 5 cm is recast into a right circular cone of height 4 cm. What is the volume of the resulting cone?

1. 12 π cm³
2. 100 π cm³
3. 33 π cm³
4. 32 π cm³
5. 4 π cm³

---

---

Video Explanation

---

---

Explanatory Answer | GRE Geometry Practice Question 1

Step 1 of solving this question: Compute Slant Height of the Resulting Cone

The sector of a circle with radius 5 cm.
When recast into a right-circular cone of height 4 cm the sector will appear as shown in the diagram.
The radius of the sector will be the slant height of the cone.
So, the slant height of the cone is 5 cm.

---

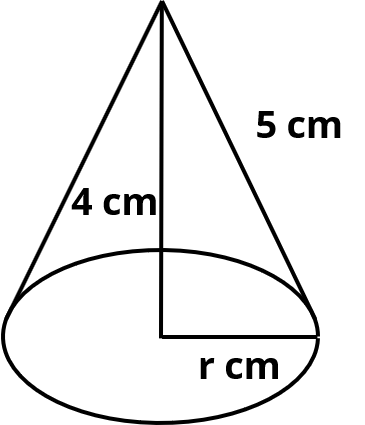
Step 2 of solving this question: Compute the base radius of the cone

The base radius, slant height, and the height of the cone form a right triangle, with the slant height being the hypotenuse of the triangle.
Slant height of the cone, which is the hypotenuse of the triangle is 5 cm.
The height of the cone, which is one of the perpendicular sides of the triangle is 4 cm.
By Pythagoras theorem, we can compute the radius r =\\sqrt{(\text{Slant Height})^2 - (\text{Height})^2})
r = \\sqrt{(5)^2 -(4)^2}) = \\sqrt{25 − 16}) = \\sqrt{9}) → r = 3 cm

---

Step 3 of solving this question: Compute the Volume of the Cone

Volume of a right circular cone = \\frac{1}{3}) π r² h
radius = 3 cm and height = 4 cm
So, volume = \\frac{1}{3}) π × 32 × 4 = 12 π cm³

Choice A is the correct answer