GRE® Select One or More Answers #5

This GRE quant practice question is a coordinate geometry problem solving question. The question is a select one or more answers type question in the GRE quantitative reasoning section. This question tests the concept of computing Slope of a Line. An interesting question to understand what values can the slope of a line take given some parameters about the line.

Question 5: Which of the following could be the slope of the line that passes through the point (4, 5) and intercepts the y-axis below the origin?
Indicate all that apply.

1. -\\frac{5}{4})
2. \\frac{5}{4})
3. \\frac{7}{4})
4. \\frac{6}{5})
5. \\frac{4}{5})
6. 2

---

---

Video Explanation

---

---

Explanatory Answer | Select One or More Answers

Step 1 : Slope of the line passing through (4, 5) and a point with a negative y-intercept

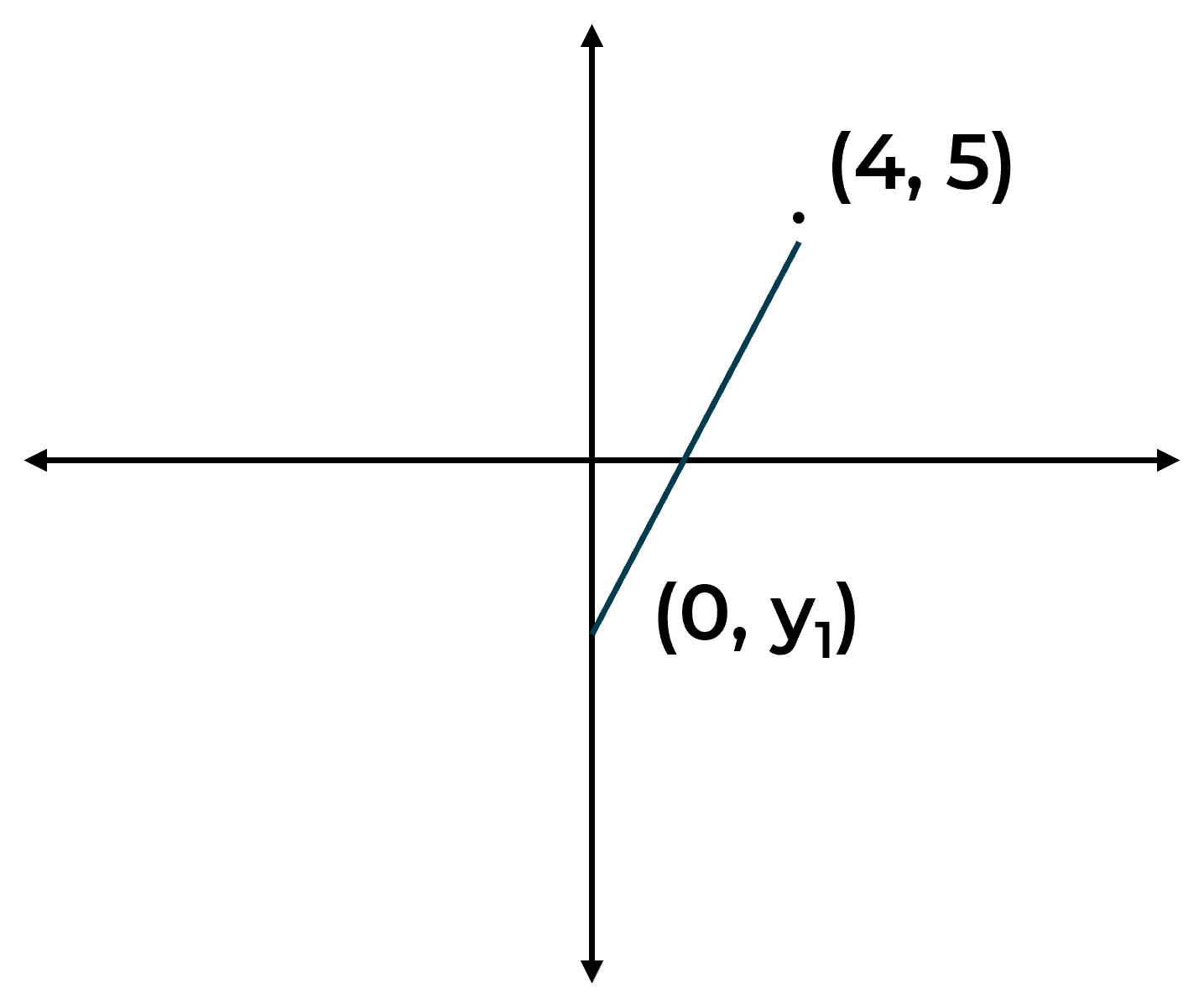
The line passes through the point (4, 5) and cuts the y-axis below the x axis.
At the point where it meets the y-axis, the x-coordinate will be 0.
The point will be of the form (0, y₁)

The slope of the line, m = \\frac{({y}_{2} - {y}_{1})}{({x}_{2} - {x}_{1})})
→ m = \\frac{(5 - {y}_{1})}{(4 - 0)}) = \\frac{5 - {y}_{1}}{4})

The y-intercept is a negative value because the line intercepts the y-axis below the x-axis.
Hence, the numerator of the above expression will take a value greater than 5.
Therefore, the slope will be greater than \\frac{\text{5}}{\text{4}})

From among the values in the five answer options, ones that have a value greater than \\frac{\text{5}}{\text{4}}) are C & F.

---

Alternative method: Slope of the line passing through the origin and (4, 5)

The slope of the line, m = \\frac{({y}_{2} - {y}_{1})}{({x}_{2} - {x}_{1})})
→ m = \\frac{\text{(5 - 0)}}{\text{(4 - 0)}}) = \\frac{\text{5}}{\text{4}})

The line that makes a negative intercept on the y-axis is going to be steeper than the line that passes through the origin.
Hence, the slope is going to be higher than \\frac{\text{5}}{\text{4}})
The options that are greater than \\frac{\text{5}}{\text{4}}) are C and F.